Log Calculator
Anti-Log Calculator
| Number ∓ Base | Amount |
|---|---|
| Enter the number(to the power of 10) | |
| Select the Base value | |
| | |
| Antilog Result | Amount |
|---|---|
| Antilog value |

| Number ∓ Base | Amount |
|---|---|
| Enter the number(to the power of 10) | |
| Select the Base value | |
| | |
| Antilog Result | Amount |
|---|---|
| Antilog value |
Calculating log (logbx):
logbx = y implies by = x.
Where,
Calculating Antilog:
y = 10x
Where,
- Guide Authored by Corin B. Arenas, published on October 1, 2019
If you've ever wondered how experts determine great distances, earthquake intensity, and global economic growth rates, then you've come to the right place.
Calculating very large sums can be slow and confusing. But with the help of logarithms (log) and antilogarithms (antilog), calculations can be made simpler.
Read on to learn more about log and antilog, how they work, and why these are relevant mathematical concepts.

A logarithm is the power to which a number (referred to as the base) must be multiplied to itself to obtain a given number. In simpler terms, logarithm solves the problem:
How many times do we multiply b to obtain another number y?
Logarithm counts the number of times the same factor must be multiplied to arrive at a given number.
Log was invented in the 16th century as a calculation tool by Scottish mathematician, physicist and astronomer, John Napier. He wrote the book Mirifici Logarithmorum Canonis Descriptio with tables and numbers discussing natural logarithms, laying down the groundwork for its basic concept.
Napier coined the term logarithm from the Greek word logos which means ‘ratio or proportion,' and arithmos which means ‘number.' When combined, it literally means ‘ratio number.'
The natural logarithm of a number is its log to the base of the constant e, where e is approximately equal to 2.718281828459. The equation is written as loge(x).
If a logarithm does not specify a base, like this example: log(1000), it's known as a common logarithm that uses the base 10.
log(1000), it's known as a common logarithm that uses the base 10.

Once you start calculating figures by millions, billions and trillions, it can get quite taxing. Whether it concerns counting a lot of money, the growth of populations, or covering large distances, log can work for you. It can simplify large sums that involve long and confusing equations, making them easier to grasp.
Here is the standard equation for log:
logb(x) = y
Where,
To understand how the concept works, here's an example with a smaller number:
Question: How many 2s do we multiply to get 32?
b = 2, x = 32
log2(32) = y
Answer: 2 x 2 x 2 x 2 x 2 = 32
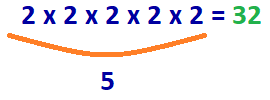
5 number 2s must be multiplied to obtain the number 32.
The answer: log2(32) = 5
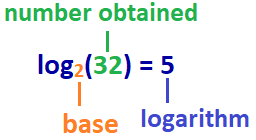
Therefore, in this example: The logarithm of 32 with base 2 is 5, or log base 2 of 32 is 5.
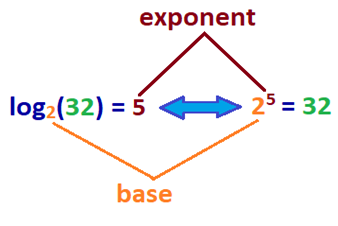
Moreover, log is the inverse function of exponentiation, where the mathematical operation is written as bn. b is the base that is multiplied according to the power of n, which is the number of times it is multiplied to itself.
What does this mean? The log of a number is the exponent to which base b is multiplied to obtain a given number. To give you a better idea, refer to the sample log equation set alongside its exponential equation below.
| log | exponent |
|---|---|
| log2(32) = 5 | 25 = 32 |
Now let's try it with a large number.
Question: How many 10s do we multiply to get 150,000,000,000?
b = 10, x = 150,000,000,000
logb(x) = y
log(150,000,000,000) = y
y = 11.1760912590557
log(150,000,000,000) = 11.1760912590557
This is solved by using the log function in a scientific calculator. Or use the calculator on this page to get the answer.

A log number can then be returned to its original number. This can be done using antilogarithm (antilog). Thus, the antilog is the inverse function of log. Likewise, antilog functions to exponentiate a simplified log value.
To compute the antilog of a number y, you must raise the logarithm base b (usually 10, sometimes the constant e) to the power that will generate the number y.
Here is the equation for antilog using base 10:
10x = y
Where x is the exponent and y is the antilog value.
For instance, if we take this equation, log(5) = x, its antilog will be 10x = 5.
Now let's try it with a larger number.
If we take log(150,000,000,000) = x, its antilog will be 10x = 150,000,000,000.

Prior to the invention of calculators, logarithms were used to simplify computations in various fields of knowledge, such as navigation, surveying, astronomy, and later on, engineering.
Imagine sailing in the middle of nowhere in the 16th century. Navigators back then relied on the position of stars and a sextant mechanism to pinpoint their exact location. Without modern technology to help you compute great distances, you can use log to simplify your calculations. Accuracy is important, or you risk more days at sea with meager supplies. Fewer equations mean less room for error.
What about other practical applications? Live Science states logarithms relate geometric progressions to arithmetic progressions. If you've ever noticed repetitive shapes and patterns in nature, architecture, and art, these formations possess their own corresponding logarithmic values.
Today, knowledge from how these patterns work influences the way humanity constructs and designs houses, buildings, and urban landscapes.
Logarithms are also used to express the extent and intensity of certain scales. Apart from wide distances, and high speeds, it measures other things such as:
Let's take decibels as an example. To make speakers louder by 10 decibels, it must be supplied by 10 times the power. As you increase it to +20 dB, it will need 100 times the power, and by +30 dB it will need 1,000 the power.
Moreover, sound intensity progresses arithmetically. It also changes proportionally with the logarithm of a sound wave which progresses geometrically.
Below is a table from Live Science listing different logarithmic scales with their corresponding linear scales.
| Field of Measurement | Linear Scale | Logarithmic Scale |
|---|---|---|
| Sound Intensity | Power (×10) | Decibels (dB) (+10) |
| Note Pitch | Frequency (×2) | Note (+12 half steps) |
| Brightness of Star | Power per unit area (×100) | Magnitude (-5) |
| Earthquake Intensity | Energy (×1000) | Richter Scale (+2) |
| Wind Intensity | Wind speed (×1.5) | Beaufort Scale (+1) |
| Mineral Hardness | Absolute hardness (×3 approx.) | Mohs Scale (+1) |
| Acidity or Basicity | Concentration of H+ions (×10) | pH (-1) |

According to Kalid Azad, the math educator behind BetterExplained.com, logarithms are how we figure out how fast something is growing.
Common logarithms basically describe numbers in terms of their powers of 10. When it comes to interest rate, the logarithm is the growth in an investment.
In determining the GDP growth rate of a country, analysts review GDP in subsequent years. They take the GDP of the previous year, and the GDP the following year, then compute the logarithm to find the estimated growth rate.
Search engines use the link graph to help score the importance, trustworthiness & authority of documents across the web. Google's PageRank was a major evolution in search which boosted search relevancy and helped Google search marketshare.
According to Azad, in a scale of 1 to 10, a landing page with a PageRank of 2 is 10 times more popular than a page with a PageRank of 1. If a site has a PageRank of 5, and a competitor site has a PageRank of 9, then it has a difference of 4 orders of magnitude.
An order of magnitude means roughly a 10x difference, or a ranking is 1 digit larger compared to the other. In this case, a site with a PageRank 9 is 100,000,000 more popular than a site with PageRank 1.
Log and antilog are significant computing methods that allow us to simplify large sums. Simplifying shortens the computation process and makes calculations easier to grasp. This helps reduce room for error.
Moreover, using log provides measurable scales for gauging natural phenomenon, like earthquake intensity, the force of windstorms, and the brightness of stars. In terms of finance, logarithms allow us to pinpoint interest rates and economic growth rates.
It's practically used in many fields. Large values that depend on the accuracy of measurement benefit from using logarithmic calculations.
Corin is an ardent researcher and writer of financial topics—studying economic trends, how they affect populations, as well as how to help consumers make wiser financial decisions. Her other feature articles can be read on Inquirer.net and Manileno.com. She holds a Master’s degree in Creative Writing from the University of the Philippines, one of the top academic institutions in the world, and a Bachelor’s in Communication Arts from Miriam College.